Exponential Growth and Decay - ppt download
In many natural phenomena, quantities grow or decay at a rate proportional to their size. Examples: The number of individuals in a population of animals or bacteria at a certain time t In nuclear physics where the mass of a radioactive substance decays at a rate proportional to the mass. In chemistry, the rate of a molecular first-order reaction is proportional to the concentration of the substance. In finance, the value of a savings account with continuously compounded interest increases at a rate proportional to that value.
Examples: The number of individuals in a population of animals or bacteria at a certain time t. In nuclear physics where the mass of a radioactive substance decays at a rate proportional to the mass. In chemistry, the rate of a molecular first-order reaction is proportional to the concentration of the substance. In finance, the value of a savings account with continuously compounded interest increases at a rate proportional to that value.
Law of natural growth (if k > 0) or the law of natural decay (if k < 0). It is called a differential equation because it involves an unknown function y and its derivative dy /dt.
Find dy/dt of any exponential function of the form y (t) = Cekt (C is constant) y (t) = C (kekt) = k (Cekt) = ky (t)
Example 2; Population Growth. Use the fact that the world population was 2560 million in 1950 and 3040 million in 1960 to model the population of the world in the second half of the 20th century. (Assume exponential). Find the relative growth rate Use the model to estimate the world population in 1993 and to predict the population in the year Let t = 0 in the year Population P (t) in millions of people. Then P (0) = 2560 and P (10) =
Find the formula P(t) given it is an exponential equation. P(t) = P (0)ekt. = 2560ekt. Find k when P(10)=3040 million. P (10) = 2560e10k =
The relative growth rate is about 1.7% per year and the model is. P(t) = 2560e t. Estimate that the world population in =43 years. P(43) = 2560e (43) 5360 million. Predict the population in 2020 will be = 70. P(70) = 2560e (70) 8524 million.
m(t) = m0ekt. Physicists express the rate of decay in terms of half-life, the time required for half of any given quantity to decay.
The half-life of radium-226 is 1590 years. (a) A sample of radium-226 has a mass of 100 mg. Find a formula for the mass of the sample that remains after t years. Solution: m(t) = mass of radium-226 (in mg) after t yrs. m(t) = m(0)ekt. = 100ekt. y(1590)= ½ (100) 100e1590k = k = = -ln (2)
m(t) = 100e (-(ln 2)t/1590) We know… eln 2 = 2. So, m(t) = 100 2(-t /1590)
(b) Find the mass after 1000 years to the nearest milligram. The mass after 1000 years is. m(1000) = 100e–(ln 2)1000/1590 65 mg.
(c) When will the mass be reduced to 30 mg Find the value of t where, m (t) = e–(ln 2)t /1590 = 30. e–(ln 2)t /1590 = 0.3.
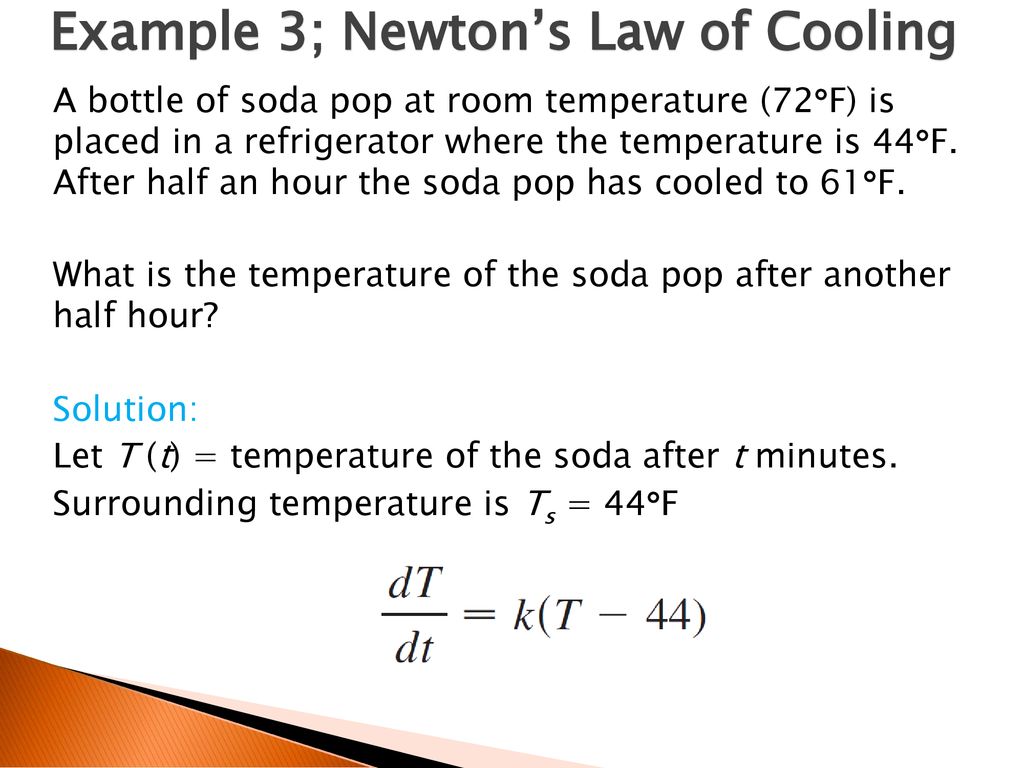
Newton’s Law of Cooling states that the rate of cooling of an object is proportional to the temperature difference between the object and its surroundings, provided that this difference is not too large. Let T (t) be the temperature of the object at time t and Ts be the temperature of the surroundings, then Newton’s Law of Cooling as a differential equation: where k is a constant.
y (t) = T (t) – Ts (Ts is constant) y (t) = T (t)
A bottle of soda pop at room temperature (72F) is placed in a refrigerator where the temperature is 44F. After half an hour the soda pop has cooled to 61F. What is the temperature of the soda pop after another half hour Solution: Let T (t) = temperature of the soda after t minutes. Surrounding temperature is Ts = 44F.
Given, T(30) = 61, y(30) = 61 – 44. y(30) = e30k = 17. k – Let y = T – 44, y (0) = T (0) – 44. y (0) = 72 – 44. y (0) = 28. y (t) = y (0)ekt. y (t) = 28ekt.
Thus, y(t) = 28e– t. T(t) = e– t. T(60) = e– (60) T(60) So after another half hour the pop has cooled to about 54 F.
The pop cools to 50F after about 1 hour 33 minutes.
Annual: 1 term. Semiannual: 2 terms. Quarterly: 4 terms. Monthly: 12 terms. Daily; 365 terms. Compounding continuously. A (t) = A0ert.
If $1000 invested for 3 years at 6% interest, find the amount of the investment that is compounded continuously. A(3) = $1000e(0.06)3 = $
Summarize Notes. Read section 3.8. Homework. Pg.242 #1,3,4,5,8,9,10,13,15,16,19,20.

PPT - Exponential Growth and Decay PowerPoint Presentation, free download - ID:5571904
Characteristics of Exponential Functions

Module 10: Lesson 10.3 Modeling Exponential Growth and Decay - ppt download
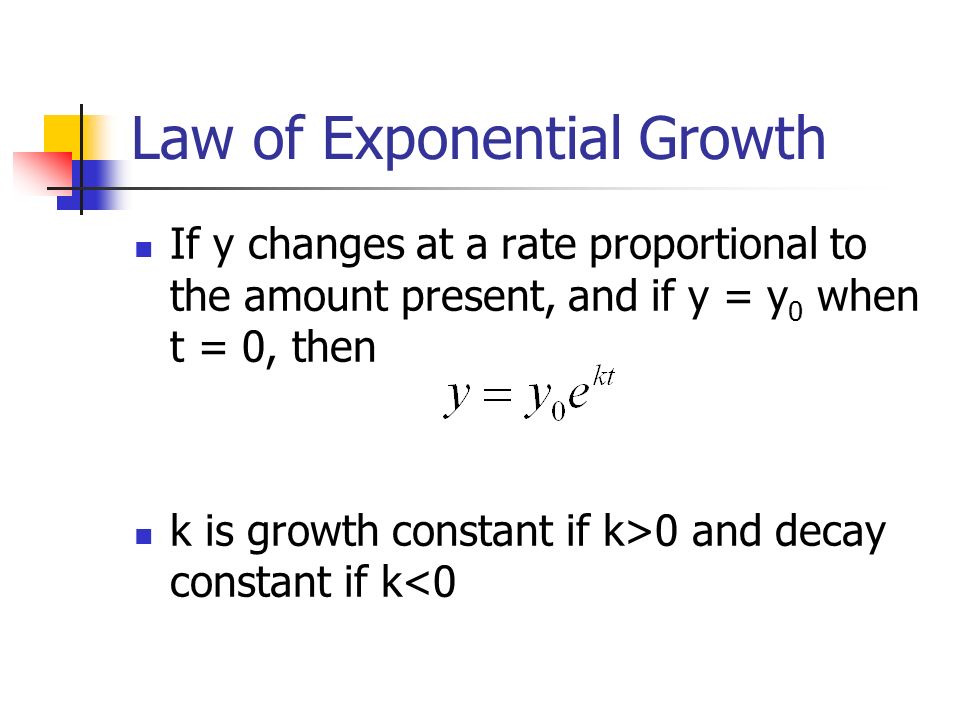
Table of Contents 5. Section 5.8 Exponential Growth and Decay. - ppt download

Exponential Growth and Decay - ppt download

6.1 Exponential Growth and Decay Functions - ppt download

8-8: E XPONENTIAL G ROWTH AND D ECAY Essential Question: Explain the difference between exponential growth and decay. - ppt download

image1./2792470/7-7-exponential-grow

5.2 Growth and Decay Law of Exponential Growth and Decay - ppt download

8-8: E XPONENTIAL G ROWTH AND D ECAY Essential Question: Explain the difference between exponential growth and decay. - ppt download

6.4 Exponential Growth and Decay - ppt download

Exponential Growth and Decay - ppt download

Exponential Function Formula

Exponential Functions: Simple Definition, Examples - Statistics How To